|
Figure 7 has been taken from
our most recent article
.
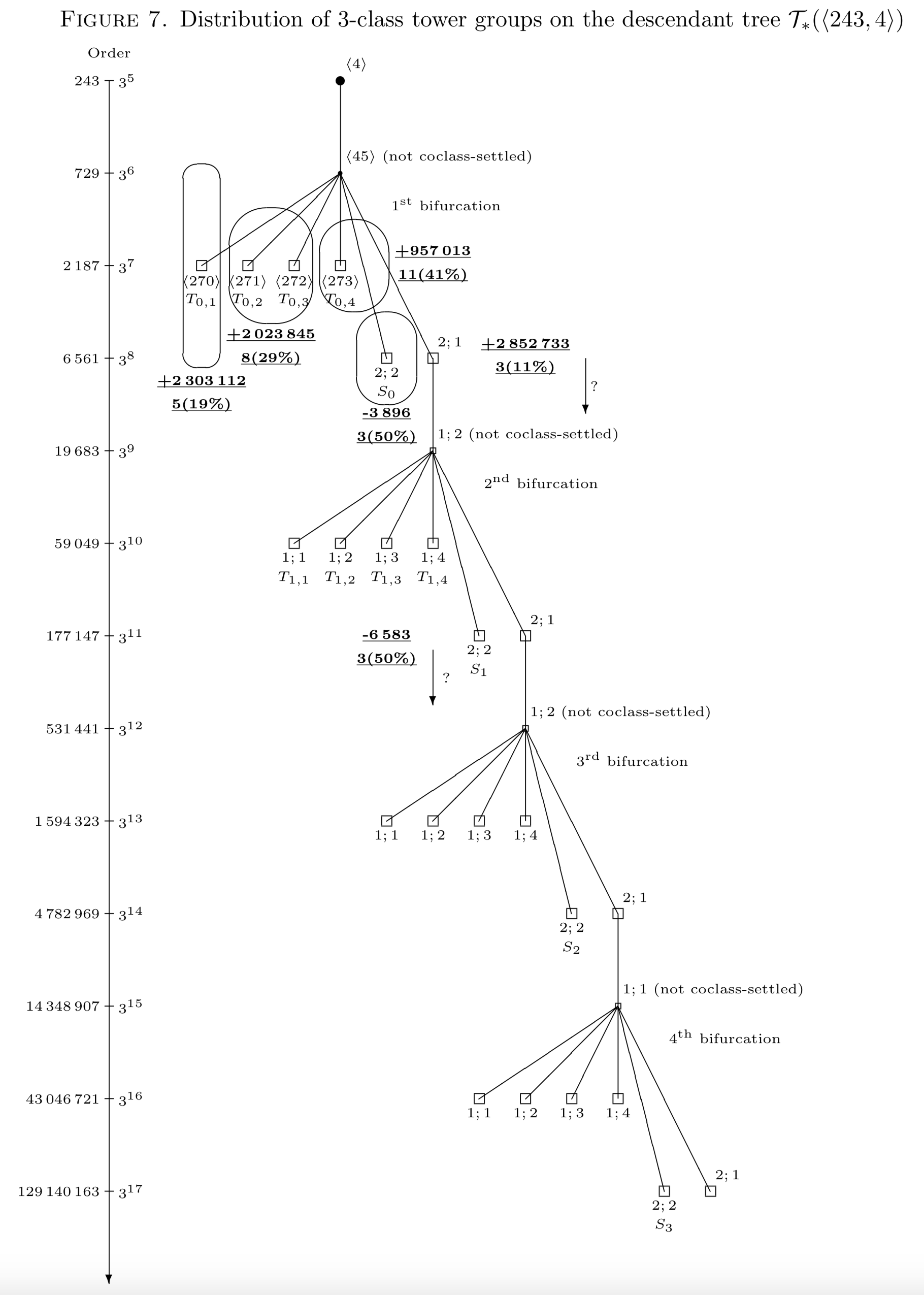
It visualizes the top region of the pruned descendant tree
of the sporadic metabelian 3-group <243,4>.
This is not a usual coclass tree with a periodic uniserial
chain of vertices on the infinite mainline.
Here we rather have strictly periodic bifurcations
occurring at every other vertex of the infinite path.
The actual infinitude has been proved indirectly by
L. Bartholdi and M.R. Bush in 2007, who constructed
an infinite subsequence (Si)i≥0 of Schur σ-groups
with indefinitely increasing coclass and derived length.
Figure 7 is an arithmetically structured tree diagram
showing the minimal discriminants and absolute frequencies
of the hits of vertices surrounded by an oval
by 3-class tower groups of real and complex quadratic fields.
A particular difficulty of the investigation of this tree is that
all vertices G share the common IPAD t(G) = ( 13, 13, 13, 21 )
and the common IPOD k(G) = (4111). For the distinction of vertices,
iterated IPADs and IPODs of second or higher order are required.
|
|
|
|

