|
Figure 8 has been taken from
our most recent article
.
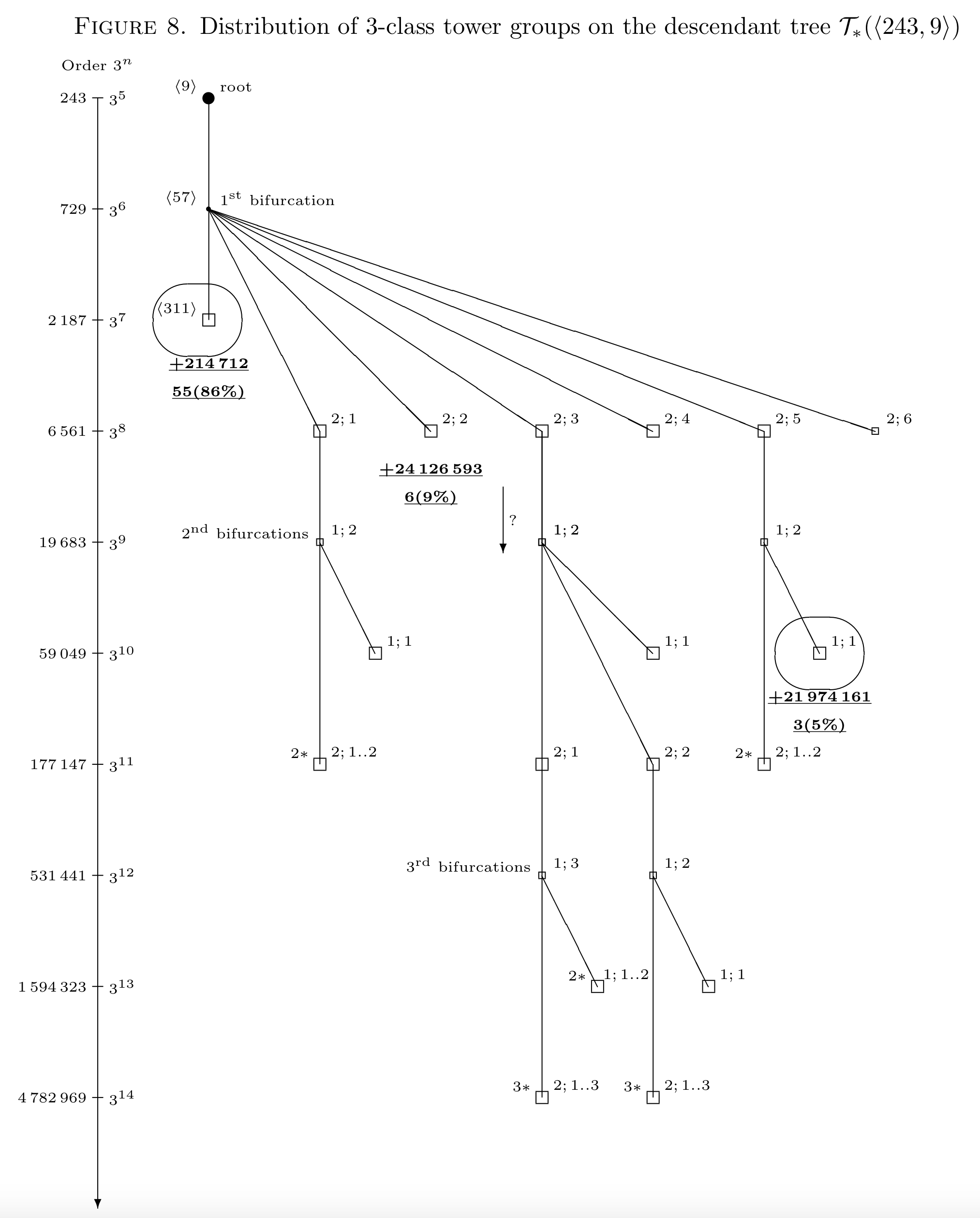
It visualizes the top region of the pruned descendant tree
of the sporadic metabelian 3-group <243,9>.
The represented subtrees with roots #2;1, #2;3, #2;5 are finite.
The subtrees with roots #2;2, #2;4, #2;6 are omitted because
they either reveal an unusual depth or complexity.
It seems to be unknown whether the entire tree is infinite or not.
Figure 8 is an arithmetically structured tree diagram
showing the minimal discriminants and absolute frequencies
of the hits of vertices surrounded by an oval
by 3-class tower groups of real quadratic fields.
A particular difficulty of the investigation of this tree is that
all vertices G share the common IPAD t(G) = ( 21, 21, 21, 21 )
and the common IPOD k(G) = (2143). For the distinction of vertices,
iterated IPADs and IPODs of second or higher order are required.
|
|
|
|

